Preliminary Result of the Mar 11, 2011 Mw 9.1 Honshu Earthquake
(March
11, 2011)
(Updated solution)
Guangfu Shao, Xiangyu Li, Chen Ji, Department of Earth Science, UCSB
Data Process
We used the broadband
waveforms automatically downloaded from the IRIS DMC to constrain the slip
history of this giant Earthquake. During this analysis, we selected 23
teleseismic broadband P waveforms, 14 broadband SH waveforms, and 41 long
period surface waves. These waveforms are converted to displacement by removing
the instrument response. We use the hypocenter of the USGS (Lon.=142.369 deg.;
Lat.=38.322 deg; Depth= 24 km).
Focal Mechanism Analysis
We used the long period
surface waves (period from 166 s to 333 s) to constrain the focal mechanism of
this giant earthquake. Our algorithm approached an earthquake source as
multiple double-couples and can automatically determine the number of point
sources by performing F test. We notice that a single pure thrust double couple
is sufficient to match the long period radiation of this earthquake (Table 1).
The low angle nodal plane inferred has a strike of 199 deg., a dip angle of 10
deg, and a slip angle of 92 deg. The total seismic moment is about 5.0x10^22
Nm, or a moment magnitude of 9.1, the largest event since the 2004 Mw 9.15
Sumatra-Andaman island earthquake.
Both the small centroid
depth (14 km) and dip angle (11 degrees) suggested that the rupture occurred
near the trench axis, consistent with the centroid location (lat=38.1 deg.,
lon=143.0 deg). It is noteworthy that the low angle fault plane is consistent
with the plate interface constrained with wide angle reflection and refraction
profile across the source region (Miura et al., 2005).
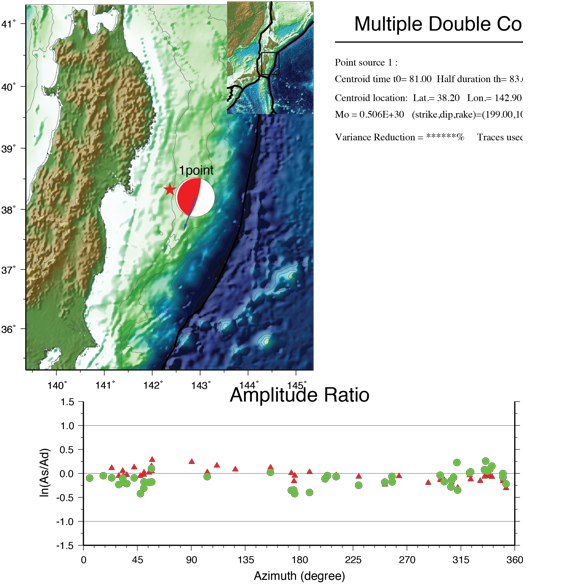
Figure
1. Ratios of peak amplitude of data (Ad) and synthetics (As). The red triangles
and green circles denote the Rayleigh and Love waves, respectively.
Finite Fault
Analysis
We define the fault plane based on our point source solution, USGS hypocenter, and trench axis. The preferred low angle fault plane orients 198. deg. north and dip 10 deg. to the west. The seismic moment release based upon this plane is 5.6 x10^22 Nm using a 1D crustal model interpolated from CRUST2.0 (Bassin et al., 2000). Most of slip occurred at shallow depth from 8 to 20 km.
Cross-section of
slip distribution

Figure 2. Cross-section of slip distribution. The strike direction of fault plane is indicated by the black arrow and the hypocenter location is denoted by a red star. The slip amplitudes at subfaults are showed in color and slip directions of the hanging wall relative to the footwall are indicated by white arrows. Contours show the rupture initiation time in sec.
Moment rate function

Figure 3. Moment rate function.
Comparison of
data and synthetic seismograms
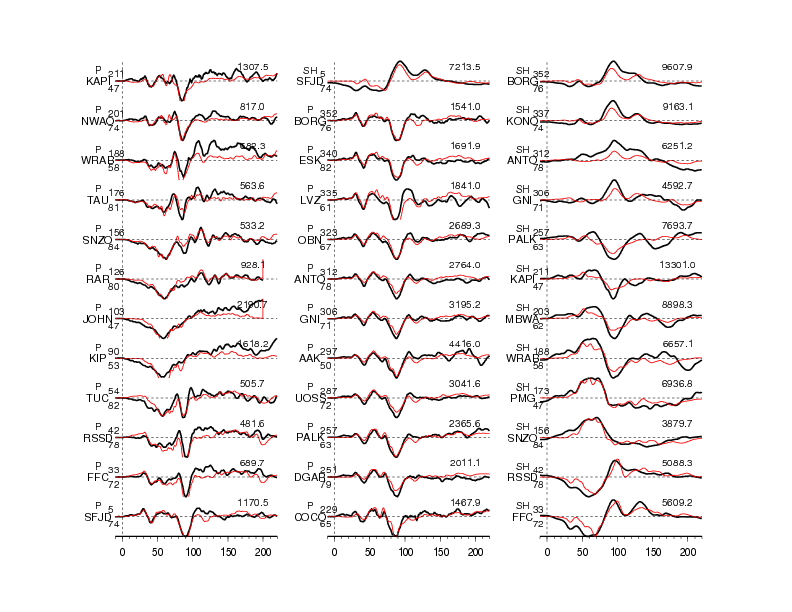
Figure 3. Comparison of teleseismic body waves. The data is shown in black and
the synthetic seismograms are plotted in red. Both data and synthetic
seismograms are aligned on the P or SH arrivals. The number at the end of each
trace is the peak amplitude of the observation in micro-meter. The number above
the beginning of each trace is the source azimuth and below is the epicentral
distance.

Figure 3.1. Comparison of long period surface waves. The data is shown in black
and the synthetic seismograms are plotted in red. Both data and synthetic
seismograms are aligned on the earthquake origin time. The number at the end of
each trace is the peak amplitude of the observation in millimeter. The number
above the beginning of each trace is the source azimuth and below is the
epicentral distance in degrees.
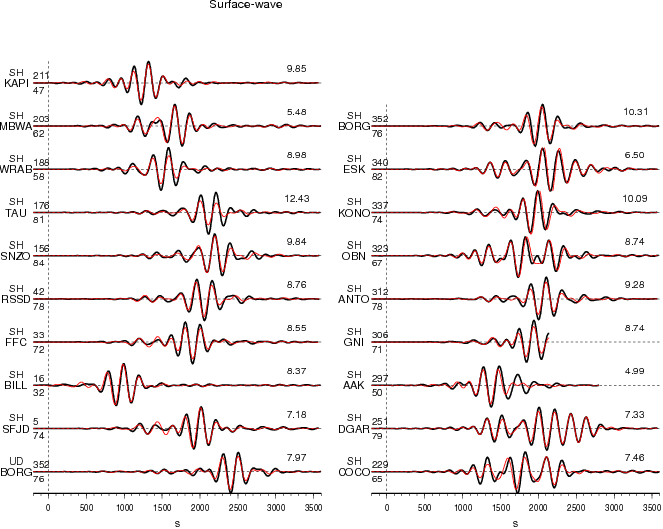
Figure 3.2. Comparison of long period surface waves. The data is shown in black
and the synthetic seismograms are plotted in red. Both data and synthetic
seismograms are aligned on the earthquake origin time. The number at the end of
each trace is the peak amplitude of the observation in millimeter. The number
above the beginning of each trace is the source azimuth and below is the
epicentral distance in degrees.
CJ's Comments:
This earthquake has relatively long rise time (16s) and slow rupture velocity (< 2 km/s). Most of its seismic moment occurred at region shallower than the hypocenter. These features are quite similar to the 2006 Mw 8.3 Kuril Islands earthquake. The peak slip is not well constrained due to the lack of high frequency in radiated body waves. But it must larger than 30 m for the given seismic moment.
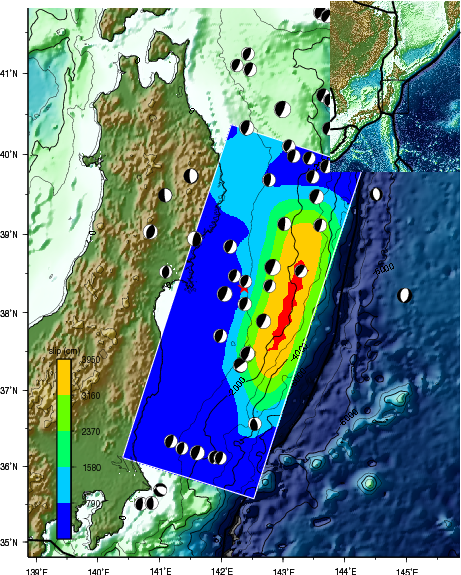
Figure 4. Surface projection of the slip distribution superimposed on ETOPO2.
The black line indicates the major plate boundary [Bird, 2003]. The GCMT focal
mechanisms of 51 magnitude 6 and larger earthquakes since 1990 are denoted as
well.
Static Displacement
Figure 5.1. Vertical
static displacement predicted based on the inverted slip model (Figure 2). Red star indicates the epicenter
location and red dots are the aftershocks (M>5) downloaded from USGS.
Figure 5.2. Horizontal
static displacement predicted based on the inverted slip model (Figure 2). Red
star indicates the epicenter location and red dots are the aftershocks (M>5)
downloaded from USGS.
Slip Distribution
|
CMTSOLUTION FORMAT |
References
Ji,
C., D.J. Wald, and D.V. Helmberger, Source description of the 1999 Hector Mine,
California earthquake; Part I: Wavelet domain inversion theory and resolution
analysis, Bull. Seism. Soc. Am., Vol 92, No. 4. pp. 1192-1207, 2002.
Bassin, C., Laske, G. and Masters, G., The Current Limits of Resolution for
Surface Wave Tomography in North America, EOS Trans AGU, 81, F897, 2000.
Acknowledgement and Contact Information
This work was supported by National Earthquake Information Center (NEIC) of United States Geological Survey. This web page is built and maintained by Dr. C. Ji at UCSB.

